Summary Notes for Entire Course

Description

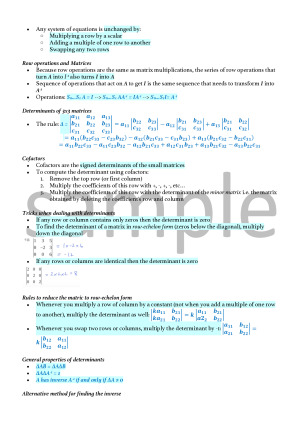
These notes thoroughly cover all lecture content from the entire course - for the final exam. Notes include but are not limited to the following topics: 2D coordinate system 3D coordinate systems 2D and 3D: Describing Lines and planes Scalars and Vectors Trigonometry Formulas Vector Components Magnitude of a Vector Representing Vectors Multiplication by a Scalar Vector Addition/Subtraction: Head-Tail Method Position Vectors Standard Basis Vectors Addition/subtraction of vectors – add/minus the components Unit vectors The Vector Dot product Work and the Dot product The Dot product Properties of the Dot product Proofs Scalar Projections Vector projections Torque and The Cross Product (aka The Vector product) Factors Affecting Torque (Γ) Cross Product - Geometrically & Properties Scalar Triple Product – Geometric Significance Equation of a line Description of a Line Segment General Equation of a plane Cartesian Equation of a plane Lines of intersection of non-parallel planes Differential Calculus assumed knowledge Continuity and differentiability Differentiation rules Product and quotient rules Chain rule Errors and small approximations, or Differentials Numerical Methods: Newton’s Method The Exponential Function The Trigonometric Hyperbolic Function Properties of the Hyperbolic Functions Derivatives of hyperbolic sine, hyperbolic cosine and the hyperbolic tangent functions Complex Numbers Complex Conjugate and Modulus Polar Coordinates Converting from Polar to Rectangular form Exponential Forms of complex numbers Exponential polar form De Moivre's Theorem Finding roots of complex numbers Inverse functions The Logarithm Function Review of log laws Logarithmic Differentiation Derivatives associated with the inverse hyperbolic functions Integrals associated with the inverse functions Derivative of complex numbers Primitive Functions Sum of Power Series Other properties of a definite integral Fundamental Theorem of Calculus Applications: using integration to find a length Applications: using integration to find a second moment of area Application: average value of a function Numerical Integration - Trapezoidal Rule Applications: using integration to find work done in the context of springs The substitute rule Change of Variable Integration by Parts Integrals in the form of ∫▒〖a polynomial/another polynomial dx〗 Integration by Partial Fractions Integrals with quadratic denominators that have no linear factors Differential Equations Number of arbitrary constants and definition of general solutions Order of A Differential Equation (DE) Linearity Solving Differential Equations: the method of "Separation of Variables" Exponential Growth and Decay Solving first order linear DES: the integrating factor method Applications Solving second order linear DEs, with constant coefficients Differentiation is linear The principle of superposition Method for solving linear second order DE's with constant coefficients Roots of the Auxiliary Equation and Associated Solution of the DE Method of Variation of Parameters (case 2 theory) Case 3 Theory Spring Mass System Model Spring model - damping Solutions of the DE: y'' + 2γy' + ω2y = 0 Inhomogeneous Case The Method of Undetermined Coefficients Matrices Rules of addition Multiplication by a scalar Matrix Operations: Matrix multiplication Identity matrix - matrix equivalent of the number 1 Transpose matrix The inverse of a 2x2 matrix Summary: rules of matrix algebra Determinants Finding the inverse of a 3x3 matrix Row operations and Matrices Determinants of 3x3 matrices Cofactors Tricks when dealing with determinants Rules to reduce the matric to row-echelon form General properties of determinants Alternative method for finding the inverse Cramer's Rule - solving equations Linear system of equations Sequences, series and applications Sequences Limit of sequences Finding limits of sequences that involve polynomials and fractions of polynomials Sigma notation and convergence of series Convergence of series by looking at partial sums Important facts about Convergence of series The ratio test: a test for convergence of series Power Series Finding intervals of convergence of power series